Efficient computation of soliton gas primitive potentials
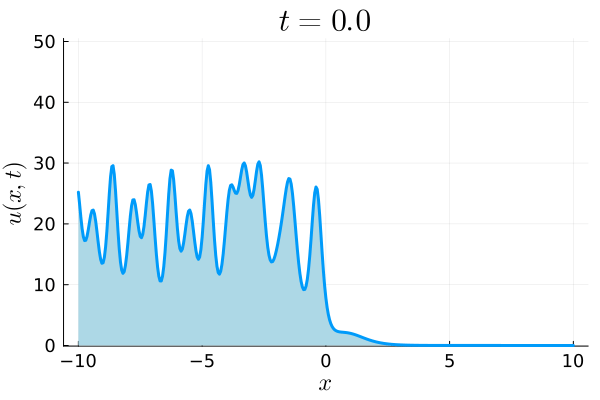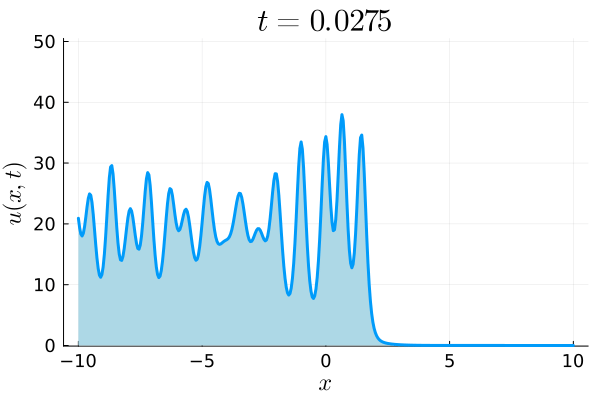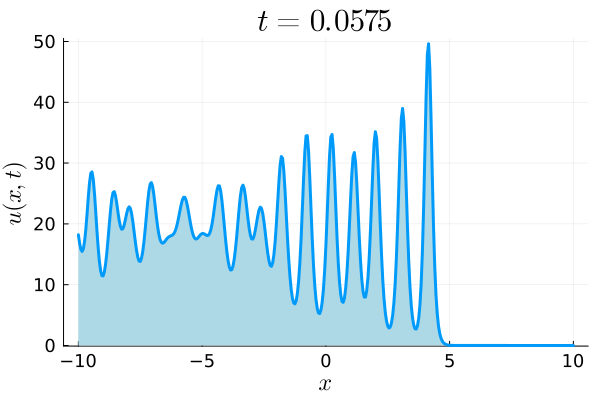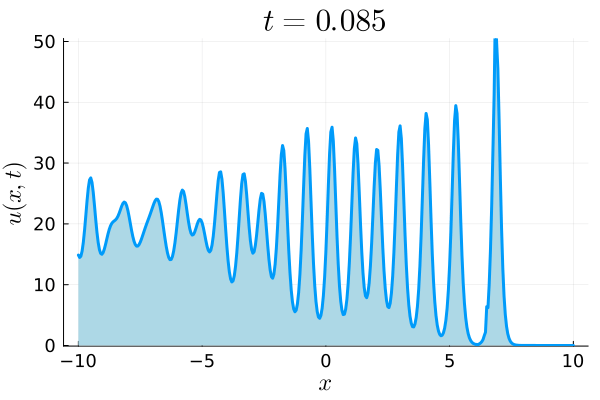 Soliton gas supported on three pairs of bands.
Soliton gas supported on three pairs of bands.
Abstract
We consider the problem of computing a class of soliton gas primitive potentials for the Korteweg-de Vries equation that arise from the accumulation of solitons on an infinite interval in the physical domain, extending to $-\infty$. This accumulation results in an associated Riemann-Hilbert problem on a number of disjoint intervals. In the case where the jump matrices have specific square-root behavior, we describe an efficient and accurate numerical method to solve this Riemann-Hilbert problem and extract the potential. The keys to the method are, first, the deformation of the Riemann-Hilbert problem, making numerical use of the so-called $g$-function, and, second, the incorporation of endpoint singularities into the chosen basis to discretize and solve the associated singular integral equation.
Type
Publication
Journal of Nonlinear Waves
This work has also resulted in the software package KdVSolitonGas.jl for Julia.